How Many Diagonals Can Be Drawn From A Fixed Vertex? (Answer)
DIAGONALS FROM FIXED VERTEX – In this article, we are going to learn about how many diagonals can be drawn from a fixed vertex.
WHAT ARE POLYGONS? – A polygon is a plane figure or shape, that has at least three straight sides, and angles. The most basic polygons we can see are triangles, squares, pentagons, and so forth.

But, how many diagonals can we draw from any fixed vertex? Simply put, we can use an equation to figure out the number of diagonals.
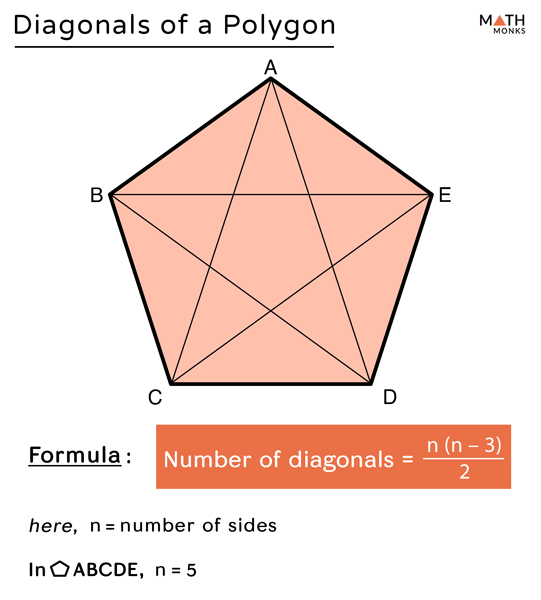
To find the total number of diagonals in a polygon, we need to multiply the number of diagonals per vertex (n – 3) by the number of vertices, n, and divide by 2 (otherwise each diagonal is counted twice).
As such we get this:

So, for a polygon with 6 sides, we can use the equation as such.
n=6. Therefore, we get 6(6-3)/2 = 9. Here is another example, this time with a polygon that has five sides.
With this, we know that n=5 and now we simply need to put it in our equation “5(5-3)/2”. Thus, the #of diagonals we can make at any vertex in a 5 sided polygon is 5.
Thanks for reading. We aim to provide our readers with the freshest and most in-demand content. Come back next time for the latest news here on Philnews.
